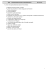Pn(x)=`£lbi(x

S U R LE T H É O R È M E D E
Par S.
MANDELBROJT,
GRACE
Clermont-Ferrand.
Soit F[f(z)] une fonctionelle linéaire dont le champ de définition est
constitué par les polynômes. Supposons que F[\] =)= 0. Si pour un polynôme de degré n, Pn{z), F[Pn(z)] = 0, ce polynôme a, au moins, un zéro dans
toute région circulaire contenant toutes les racines de Fn(x) = F[(z—x)n] = 0.
La démonstration de ce théorème est basée sur le lemme suivant:
n
Le polynôme Qn{x) = S^bi(x—aì)n
a, au moins, un zéro dans toute
région circulaire contenant tous les ai.
Pour le démontrer il suffit de remarque qu'en posant x =
Qn(x) = (p(t), on a
d[( (t)
P ^~a^
t—ai
= ^'/(f—fl'.-)"- 1 ; et le théorème est vrai
pour n, s'il est vrai pour n—1, en vertu du théorème de Lucas—Gauss.
Or il est vrai pour « = 1 .
Pour passer du lemme au théorème on remarquera qu'on peut, lorsque
les hypothèses du théorème sont réalisées, écrire:
n
Pn(x)='£lbi(x-aiy,
où les ai sont des zéros de Fn(x).
Le lemme est une certaine forme du théorème connu de Grace. Le
théorème et le lemme sont, d'ailleurs, équivalents.
NOTE ON SOME ADDITIVE
OF I N T E G E R S
By
PAUL ERDÖS,
PROPERTIES
Manchester.
i. It is well known that for suitable n's both the equations n = x2—y2
c
i
2j
2
l sl en
and n=x ry
have more than n ° °
solutions. I show that for suitable
«'s the number of solutions of the equations n=p2—q2 resp. n=p2 + q2
(P>
34
a
primes) is greater than nÎOël°ën.
I sketch the proof for n=p2—q2.
Let A = 2'3- • • pr, the product of consecutive primes, be sufficiently
large. By elementary method we prove that the number of solutions of
the congruence p2—q2=0
(mod A) with 0<q<lp<.A
is greater than
l
1+7
A 4 1 ° s > g i . But the integers of the form p2—q2
with 0<q<p<A
lie
all between 0 and A2, hence there exists a multiple of A say
n«A2)
such that the number of solutions of the equation n=p2—q2 is greater than
^ 4 log log A ^
n8
log log n_
The proof for n=p2 + q2 is much more complicated but also elementary.
It requires Brun's method.
2. Schnirelmann proved that there exists a constant c& such that
every integer is the sum of cs or less primes. Some time ago HeilbronnLandau-Scherk proved that c& < i 7 1 . By Brun's method I proved that there
exists a constant c4 such that any integer is the sum of cà or less positive
and negative squares of primes. T h e same result holds for any powers
of primes. It can be proved also that the density of integers of the form
p2 + q2—r2—s2 is positive.
3. Now I sketch some new results of N. P. Romanoff (Tomsk).
Let us denote by f(x1}x2,
• • • , xkl; y^y^, • • *JV/c2 \n) t n e number of
integers not exceeding n belonging to the sequence x-t, yj and Xi+yj, It
is an old and most important problem of the additive theory of numbers
to determine the value o f / for given Xi and yj. But this can be solved
only for special sequences. Romanoff deduced 4 formulas for the mean
value of f for general sequences of integers.
First mean-value-theorem:
2
/(*i'**>'-,*kx;yltyit• • • ,^21n)
1^X1<JC2<.
.<xkl^n
n—k9
=«c;-sc
n-\-yz+z
k
z—\
where C£ = \k)
and yz
denotes the complementary sequence of yz.
Second mean-value-theorem :
n
n
n
x
kl = l
n-kq
= nk + l—
^(n—l—y2
+ z)ki.
35
Third mean-value-theorem:
y]
/ U n x2, • • • *kX ; yltyZi
J)
—
nCn cnk — wcn
~n{-k {1
2
1 +
1
ü
rnfc +1 -t-4 -ürf c"
1+1
2
ü
• • • jv*Ä | n)
n - f c -t-1
rfc
*
a
Fourth mean-value-theorem:
[-1
=«c;-2c; 2 -c l + 2 w ^
+
f-1
2H.(,+2f„)^1J
with £n = 0 if « even, and En=i if n odd.
T h e proof depends on elementary combinatorie methods.
MODULES
Par
RÉCIPROQUES
MARCEL RIESZ, Lund.
Soit M un module constitué de certains points (vecteurs) x de l'espace
à n dimensions. L'ensemble des points (vecteurs) v tels que le produit
scalaire (x, v) soit entier pour chaque x, forme un module fermé, c.-à-d.
un module qui contient tous ses points limites. En désignant ce module
par M~l, on a la relation fondamentale
d)
(M-*rl=[M],
où [M] désigne la fermeture de M. Dans le cas où M est fermé lui-même,
cette relation se réduit à une vraie relation de réciprocité, savoir à
(M~l)~
=M. En retournant au cas général et en désignant par Nun second
module, formons au sens d e l'addition vectorielle le module M+N. O n
a alors
(2)
(M+N)~l = M-lN-1,
le second membre signifiant d'après une notation usuelle l'ensemble des
points communs à M~l et à AT—1.
Les théorèmes de Kronecker sur les approximations diophantiques sont
des conséquences évidentes des relations (1) et (2).
Soit maintenant M un module discret (c.-à-d. un grillage de points)
du rang n. Il en sera alors de même du module réciproque M~l. P a r
36